Exercise 20.1
Question 1: Which of the following are closed curves? Which of them are simple?
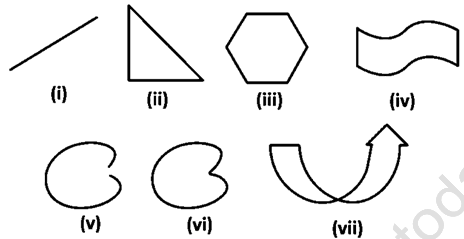
Solution 1:
Closed Curves:- (ii), (iii), (iv), (vi) and (vii)
Simple Closed Curves:- (ii), (iii), (iv) and (vi)
Question 2: Define perimeter of a closed figure.
Solution 2: Perimeter is the sum of lengths of all the sides of a closed figure.
Question 3: Find the perimeter of each of the following shapes:
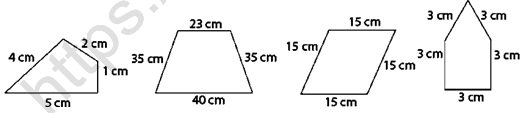
Solution 3:
Perimeter is the sum of lengths of all the sides:-
(i) Perimeter = 4 + 2 + 1 + 5 = 12 cm
(ii) Perimeter = 23 + 35 + 40 + 35 = 133 cm
(iii) Perimeter = 15 + 15 + 15 + 15 = 60 cm
(iv) Perimeter = 3 + 3 + 3 + 3 + 3 = 15 cm
Exercise 20.2
Question 1: Find the perimeters of the rectangles whose lengths and breadths are given below:
(i) 7 cm, 5 cm
(ii) 5 cm, 4 cm
(iii) 7.5 cm, 4.5 cm
Solution 1:
(i) Perimeter of a Rectangle = 2 (L+B)
Length = 7 cm
Breadth = 5 cm
Perimeter of a rectangle = 2 (7 + 5)
Perimeter of a rectangle = 2 × 12
Perimeter of a rectangle = 24 cm
(ii) Perimeter of a Rectangle = 2 (L+B)
Length = 5 cm
Breadth = 4 cm
Perimeter of a rectangle = 2 (5 + 4)
Perimeter of a rectangle = 2 × 9
Perimeter of a rectangle = 18 cm
(iii) Perimeter of a Rectangle = 2 (L+B)
Length = 7.5 cm
Breadth = 4.5 cm
Perimeter of a rectangle = 2 (7.5 + 4.5)
Perimeter of a rectangle = 2 × 12
Perimeter of a rectangle = 24 cm
Question 2: Find the perimeters of the squares whose sides are given below:
(i) 10 cm
(ii) 5 m
(iii) 115.5 cm
Solution 2:
(i) Perimeter of a square = 4 × Side
Length = 10 cm
Perimeter of a square = 4 × 10
Perimeter of a square = 40 cm
(ii) Perimeter of a square = 4 × Side
Length = 5 m
Perimeter of a square = 4 × 5
Perimeter of a square = 20 m
(iii) Perimeter of a square = 4 × Side
Length = 115.5 cm
Perimeter of a square = 4 × 115.5
Perimeter of a square = 462 cm
Question 3: Find the side of the square whose perimeter is:
(i) 16 m
(ii) 40 cm
(iii) 22 cm
Solution 3:
(i) Perimeter of Square = 4(Side)
16m = 4(Side)
Side= 16m/4
Side = 4m
Hence, the side of the square = 4m
(ii) Perimeter of Square = 4(Side)
40m = 4(Side)
Side= 40m/4
Side = 10m
Hence, the side of the square = 10m
(iii) Perimeter of Square = 4(Side)
22m = 4(Side)
Side= 22m/4
Side = 5.5m
Hence, the side of the square = 5.5m
Question 4: Find the breadth of the rectangle whose perimeter is 360 cm and whose length is
(i) 116 cm
(ii) 140 cm
(iii) 102 cm
Solution 4:
(i) Perimeter of a Rectangle = 2(L+B)
Perimeter of Rectangle = 360cm
Breadth of Rectangle = 116cm
360 = 2(L+116)
360/2 = (L+116)
180=L+116
L=180-116
L=64
Hence, the breadth of the rectangle is 64 cm.
(ii) Perimeter of a Rectangle = 2(L+B)
Perimeter of Rectangle = 360cm
Breadth of Rectangle = 140cm
360 = 2(L+140)
360/2 = (L+140)
180=L+140
L=180-140
L=40
Hence, the breadth of the rectangle is 40 cm.
(iii) Perimeter of a Rectangle = 2(L+B)
Perimeter of Rectangle = 360cm
Breadth of Rectangle = 102cm
360 = 2(L+102)
360/2 = (L+102)
180=L+102
L=180-102
L=78
Hence, the breadth of the rectangle is 78 cm.
Question 5: A rectangular piece of lawn is 55 m wide and 98 m long. Find the length of the fence around it.
Solution 5:
Breadth = 55 m
Length = 98 m
Perimeter of lawn = 2 (L+B)
Perimeter of lawn = 2 (98+55)
Perimeter of lawn = 2 × 153
Perimeter of lawn = 306m
Therefore, the length of the fence around the lawn is 306 m.
Question 6: The side of a square field is 65 m. What is the length of the fence required all around it?
Solution 6:
Side of a square field = 65 m
Perimeter of Square = 4(Side)
Perimeter of square field = 4 × side of the square
Perimeter of square field = 4 × 65
Perimeter of square field = 260 m
Therefore, the length of the fence required all around is 260 m.
Question 7: Two sides of a triangle are 15 cm and 20 cm. The perimeter of the triangle is 50 cm. What is the third side?
Solution 7:
First side of triangle will be = 15cm
Second side of triangle will be = 20cm
Let the third side will be x.
Perimeter of Triangle = Side + Side + Side
50cm=15cm+20cm+x
50cm=35cm+x
x=50cm-35cm
x=15cm
Therefore, the length of third side is 15 cm.
Question 8: A wire of length 20 m is to be folded in the form of a rectangle. How many rectangles can be formed by folding the wire if the sides are positive integers in metres?
Solution 8:
Length of wire = 20m
Perimeter = 20m
2 (L + B) = 20m
L + B = 10m
If the length of rectangle is 1m then Breadth is 9m.
If the length of rectangle is 2m then Breadth is 8m.
If the length of rectangle is 3m then Breadth is 7m.
If the length of rectangle is 4m then Breadth is 6m.
If the length of rectangle is 5m then Breadth is 5m.
Thus, five rectangles can be formed using 20m wire.
Question 9: A square piece of land has each side equal to 100 m. If 3 layers of metal wire has to be used to fence it, what is the length of the wire needed?
Solution 9:
Side of a square field = 100 m
Perimeter of square = 4(Side)
Perimeter of field = 4 × side of a square field
Perimeter of field = 4 × 100
Perimeter of field = 400m
Length of wire for 3 layers fence is = 3 × 400m
Length of wire for 3 layers fence is = 1200m
Thus, Length of wire needed for 3 layers fence is 1200 m.
Question 10: Shikha runs around a square of side 75 m. Priya runs around a rectangle with length 60 m and breadth 45 m. Who covers the smaller distance?
Solution 10:
Shikha runs around a square of side = 75m
Perimeter of the square field = 4 × 75
Perimeter of the square field = 300m
Length = 60m
Breadth = 45m
Distance covered can be found from the perimeter = 2 (L + B)
Perimeter = 2 (60+45)
Perimeter = 2 × 105
Perimeter = 210m
Thus, Priya covers the smaller distance of 210 m.
Question 11: The dimensions of a photographs are 30 cm × 20 cm. What length of wooden frame is needed to frame the picture?
Solution 11:
Dimensions of photographs = 30 cm × 20 cm
Perimeter of the photograph = 2 (L + B)
Perimeter of the photograph = 2 (30 + 20)
Perimeter of the photograph = 2 × 50
Perimeter of the photograph = 100 cm
Thus, length of the wooden frame for picture is 100 cm.
Question 12: The length of a rectangular field is 100 m. If the perimeter is 300 m, what is its breadth?
Solution 12:
Length of a rectangular field = 100m
Perimeter of a rectangular field = 300 m
Perimeter = 2 (L+B)
300m=2 (100+B)
300m/2=100+B
150=100+B
B=150-100
B=50
Hence, the breadth of the rectangular field is 50 m.
Question 13: To fix fence wires in a garden, 70 m long and 50 m wide, Arvind bought metal pipes for posts. He fixed a post every 5 metres apart. Each post was 2 m long. What is the total length of the pipes he bought for the posts?
Solution 13:
Length of the garden = 70 m
Breadth of the garden = 50 m
Perimeter of the garden = 2(L+B)
Perimeter of the garden = 2 (70 + 50)
Perimeter of the garden = 2 × 120
Perimeter of the garden = 240m
Number of posts required = 240/5
Number of posts required = 48
Length of each post = 2 m
Total length of the pipe = 48 × 2
Total length of the pipe = 96 m
Thus, the total length of the pipes is 96 m.
Question 14: Find the cost of fencing a rectangular park of length 175 m and breadth 125 m at the rate of Rs 12 per meter.
Solution 14:
Length of the rectangular park = 175 m
Breadth of the rectangular park = 125 m
Perimeter of the rectangle = 2 (L+B)
Perimeter of the rectangular park = 2 (175 + 125)
Perimeter of the rectangular park = 2 × 300
Perimeter of the rectangular park = 600 m
The cost of fencing = Rs. 12 per meter
Total cost of fencing = 12 × 600
Total cost of fencing = Rs. 7200
Thus, the cost of fencing a rectangular park is Rs 7200.
Question 15: The perimeter of a regular pentagon is 100 cm. How long is each side?
Solution 15:
Pentagon having 5 sides of same length.
Perimeter of a regular pentagon = 100 cm
Perimeter of Pentagon = 5(Side)
100m = 5(Side)
Side of the pentagon = 100/5
Side of the pentagon = 20 cm
Thus, the side of the pentagon is 20 cm.
Question 16: Find the perimeter of a regular hexagon with each side measuring 8 m.
Solution 16:
Hexagon has six sides of same length.
Side of hexagon = 8m
Perimeter of the hexagon = 6 (side)
Perimeter of the hexagon = 6 × 8
Perimeter of the hexagon = 48m
Thus, the perimeter of a hexagon is 48 m.
Question 17: A rectangular piece of land measure 0.7 km by 0.5 km. Each side is to be fenced with four rows of wires. What length of the wire is needed?
Solution 17:
Measure of rectangular piece of land = 0.7 km × 0.5 km
Perimeter of rectangle = 2 (L + B)
Perimeter piece of land = 2 (0.7 + 0.5)
Perimeter piece of land = 2 × 1.2
Perimeter piece of land = 2.4km
Length of wire needed to fence the land with 4 rows of wire = 4 × 2.4
Length of wire needed to fence the land with 4 rows of wire = 9.6km
Thus, the length of wire for fence the land is 9.6 km.
Question 18: Avneet buys 9 square paving slabs, each with a side of ½ m. He lays them in the form of a square.
(i) What is the perimeter of his arrangement?
(ii) Shari does not like his arrangement. She gets him to lay them out like a cross. What is the perimeter of her arrangement?
(iii) Which has greater perimeter?
(iv) Avneet wonders, if there is a way of getting an even greater perimeter. Can you find a way of doing this? (The paving slabs must meet along complete edges they cannot be broken)
Solution 18:
(i) Length of side of the slab = 1/2 m
One side of the square is formed by three slabs in a square arrangement
Length of side = 3 × 1/2 m = 3/2 m
The perimeter of the square arrangement = 4 × 3/2 m
The perimeter of the square arrangement = 6 m
(ii) By cross arrangement has 8 sides which form periphery of the arrangement and measure 1 m each.
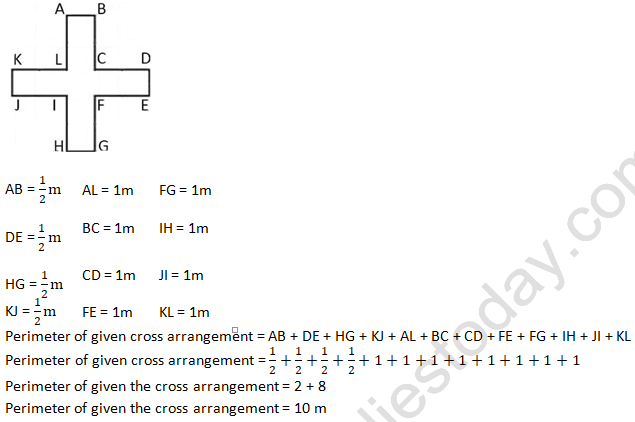
(iii) Perimeter of cross arrangement = 10 m
Perimeter of square arrangement = 6 m
Thus, Shari’s figure has greater perimeter than the perimeter Avneet’s figure of square arrangement.
(iv) Avneet cannot arrange the slabs having perimeter more than 10 m.
Exercise 20.3
Question 1: The following figures are drawn on a squared paper. Count the number of squares enclosed by each figure and find its area, taking the area of each square as 1cm2. (Fig. 20.25).
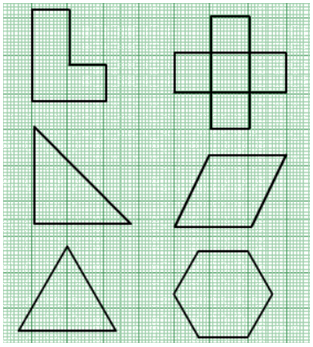
Solution 1:
(i) It is given that
Shape covers 16 complete squares.
One square = 1cm2
Area of the given shape = 16 × 1
Area of the given shape = 16cm2
(ii) It is given that
Shape covers 36 complete squares.
One square = 1cm2
Area of the given shape = 36 × 1
Area of the given shape = 36cm2
(iii) It is given that
Complete Squares = 15
Half squares = 6× 1/2=3
Shape covers 15 + 3 = 18 complete squares.
One square = 1cm2
Area of the given shape = 18 × 1
Area of the given shape = 18cm2
(iv) It is given that
Complete Squares = 20
Half squares = 8× 1/2=4
Shape covers 20 + 4 = 24 complete squares.
One square = 1cm2
Area of the given shape = 24 × 1
Area of the given shape = 24cm2
(v) It is given that
Complete Squares = 13
More than half squares = 8
Less than half squares = 7
Shape covers 13 + 8 = 21 complete squares.
One square = 1cm2
Area of the given shape = 21 × 1
Area of the given shape = 21cm2
(vi) It is given that
Complete Squares = 8
More than half squares = 6
Less than half squares = 4
Shape covers 8 + 6 = 14 complete squares.
One square = 1cm2
Area of the given shape = 14 × 1
Area of the given shape = 14cm2
Question 2: On a squared paper, draw (i) a rectangle, (ii) a triangle (iii) any irregular closed figure. Find the approximate area of each by counting the number of squares complete, more than half and exactly half.
Solution 2:
(i) Rectangle
Complete Squares = 18
Area of one square = 1cm2
Area of the rectangle = 18 × 1
Area of the rectangle = 18cm2
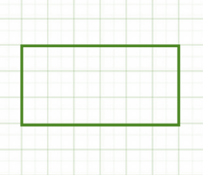
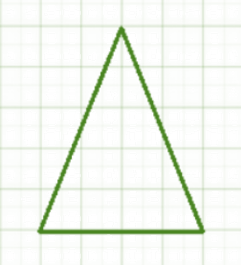
(ii) Triangle
Complete Squares = 4
More than half = 6
Less than half = 6
Total Number Squares = 4 + 6 = 10
Area of one square = 1cm2
Area of the square = 10 × 1cm2
Area of the square = 10 cm2
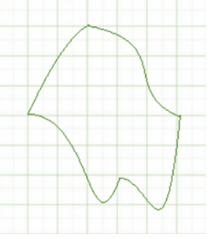
(iii) Irregular figure
Complete Squares = 10
Half Square = 1 × 1/2 = 1/2 = 0.5
More than half = 7
Less than half = 6
Total Number Squares = 10 + 7 + 0.5 = 17.5
Area of one square = 1cm2
Area of the square = 17.5 × 1cm2
Area of the square = 17.5 cm2
Question 3: Draw any circle on the graph paper. Count the squares and use them to estimate the area of the circular region.
Solution 3:

Complete Squares = 21
More than half = 15
Less than half squares = 8
Total Number Squares = 21 + 15 = 36
Area of one square = 1cm2
Area of the circle = 36 × 1cm2
Area of the circle = 36cm2
Question 4: Use tracing paper and centimetre graph paper to compare the areas of the following pairs of figures:

Solution 4:
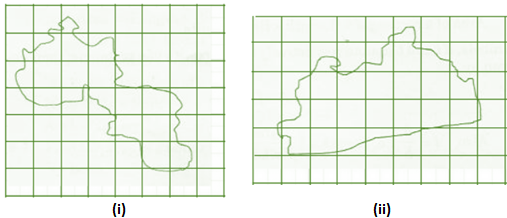
(i) Complete square = 4
More than half = 9
Less than half squares = 9
Total Number Squares = 4 + 9 = 13
Area of one square = 1cm2
Area of the shape = 13 × 1cm2
Area of the shape = 13 cm2
(ii) Complete square = 8
More than half = 11
Less than half squares = 10
Total Number Squares = 8 + 10 = 19
Area of one square = 1cm2
Area of the shape = 19 × 1cm2
Area of the shape = 19 cm2
Hence, Area of second figure has greater than that of figure (i).
Exercise 20.4
Question 1: Find the area of a rectangle, whose
(i) Length = 6 cm, breadth = 3 cm
(ii) Length = 8 cm, breadth = 3 cm
(iii) Length = 4.5 cm, breadth = 2 cm.
Solution 1:
(i) Area of a rectangle = L × B
Length = 6 cm
Breadth = 3 cm
Area of a rectangle = 6 × 3
Area of a rectangle = 18 cm2
(ii) Area of a rectangle = L × B
Length = 8 cm
Breadth = 3 cm
Area of a rectangle = 8 × 3
Area of a rectangle = 24 cm2
(iii) Area of a rectangle = L × B
Length = 4.5 cm
Breadth = 2 cm
Area of a rectangle = 4.5 × 2
Area of a rectangle = 9 cm2
Question 2: Find the area of a square whose side is:
(i) 5 cm
(ii) 4.1 cm
(iii) 5.5 cm
(iv) 2.6 cm
Solution 2:
(i) Area of a square = (Side)2
Side of a square = 5 cm
Area of the square =
Area of the square = 25 cm2
(ii) Area of a square = (Side)2
Side of a square = 4.1 cm
Area of the square =
Area of the square = 16.81 cm2
(iii) Area of a square = (Side)2
Side of a square = 5.5 cm
Area of the square =
Area of the square = 30.25 cm2
(iv) Area of a square = (Side)2
Side of a square = 2.6 cm
Area of the square =
Area of the square = 6.76 cm2
Question 3: The area of a rectangle is 49 cm2 and its breadth is 2.8cm. Find the length of the rectangle.
Solution 3:
Area of a rectangle = 49 cm2
Breadth = 2.8 cm
Area of a rectangle = L × B
49 = L × 2.8
L = 49/2.8
L = 17.5cm
Thus, the length of the rectangle is 17.5 cm.
Question 4: The side of a square is 70 cm. Find its area and perimeter.
Solution 4:
Side of a square = 70 cm
Area of a square = (side)2
Area of a square = (70)2
Area of a square = 70 × 70
Area of a square = 4900 cm2
Perimeter of a square = 4 × side
Perimeter of a square = 4 × 70
Perimeter of a square = 280 cm
Hence, the area of square is 4900 cm2 and the perimeter of square is 280 cm.
Question 5: The area of a rectangle is 225 cm2 and its one side is 25 cm, find its other side.
Solution 5:
Area of a rectangle = 225cm2
Let the given one side will be Length = 25cm and breadth will be xcm
Area of a rectangle = L × B
225 = 25 × x
x = 225/25
x = 9cm
Thus, the breadth of the rectangle is 9cm.
Question 6: What will happen to the area of rectangle if its
(i) Length and breadth are trebled
(ii) Length is doubled and breadth is same
(iii) Length is doubled and breadth is halved.
Solution 6:
(i) If we treble the Length and breadth of the rectangle.
Let length and breadth will be l and b respectively.
Area of rectangle = l × b
New length will be = 3l
New breadth will be = 3b
New area of rectangle = 3l × 3b
New area of rectangle = 9lb
Thus, the area of the rectangle becomes 9 times more than its original area.
(ii) If we double the length and breadth is same.
Let length and breadth will be l and b respectively.
Area of rectangle = l × b
New length = 2l
New breadth = b
New area of the rectangle = 2l × b = 2lb
Thus, the area of the rectangle becomes 2 times more than the original area.
(iii) If we double the length and breadth is halved.
Let length and breadth will be l and b respectively.
Area of rectangle = l × b
New length = 2l
New breadth = b/2
New area of the rectangle = 2l × b/2 = lb
Thus, the area of the rectangle does not change.
Question 7: What will happen to the area of a square if its side is:
(i) Tripled
(ii) Increased by half of it.
Solution 7:
(i) Tripled
Let the side will be s.
Area of square = (side)2
New side = 3s
Area of square = (3s)2
Area of square = 3s × 3s
Area of square = 9s2
Thus, the area is 9 times more than original area.
Question 8: Find the perimeter of a rectangle whose area is 500cm2 and breadth is 20cm.
Solution 8:
Area of the rectangle = 500cm2
Breadth of the rectangle = 20cm
Area of rectangle = L × B
500 = L × 20
L = 500/20
L = 25cm
Perimeter of rectangles = 2 (L + B)
Perimeter of rectangles = 2 (25 + 20)
Perimeter of rectangles = 2 × 45
Perimeter of rectangles = 90 cm
Thus, the perimeter of the rectangle is 90 cm.
Question 9: A rectangle has the area equal to that of a square of side 80 cm. If the breadth of the rectangle is 20 cm, find its length.
Solution 9:
Side of a square = 80 cm
Area of the square = (side)2
Area of square = (80)2
Area of square = 80 × 80
Area of square = 6400 cm2
Area of rectangle = Area of square = 6400 cm2
Breadth = 20 cm
Area of rectangle = L × B
6400 = L × 20
L = 6400/20
L = 320 cm
Thus, the length of the rectangle is 320 cm.
Question 10: Area of a rectangle of breadth 17 cm is 340 cm2. Find the perimeter of the rectangle.
Solution 10:
Breadth = 17 cm
Area of rectangle = 340 cm2
Area of rectangle = L × B
340 = L × 17
L = 340/17
L = 20cm
Perimeter of a rectangle = 2 (L + B)
Perimeter of a rectangle = 2 (20 + 17)
Perimeter of a rectangle = 2 × 37
Perimeter of a rectangle = 74cm
Thus, the perimeter of the rectangle is 74 cm.
Question 11: A marble tile measures 15 cm × 20 cm. How many tiles will be required to cover a wall of size 4 m × 6 m?
Solution 11:
Marble tile’s Measure = 15cm × 20cm
Size of wall = 4m × 6m = 4 × 100cm × 6 × 100cm = 400cm × 600cm
Area of tile = 15 cm × 20 cm = 300 cm2
Area of wall = 400 cm × 600 cm = 240000 cm2
Number of tiles = (Area of wall)/(Area of one tile)
Number of tiles = 2,40,000/300
Number of tiles = 800 tiles
Thus, 800 tiles are required to cover a wall.
Question 12: A marble tile measures 10 cm × 12 cm. How many tiles will be required to cover a wall of size 3 m × 4 m? Also, find the total cost of the tiles at the rate of Rs 2 per tile.
Solution 12:
Marble tile’s Measure = 10cm × 12cm
Size of the wall = 3m × 4m = 3 × 100cm × 4 × 100cm = 300cm × 400cm
Area of marble tile = 10cm × 12cm = 120cm2
Area of wall = 300cm × 400cm = 120000 cm2
Number of tiles = (Area of wall)/(Area of one tile)
Number of tiles = 120000/120
Number of tiles = 1000 tiles
Cost of one tile = Rs. 2
Cost of 1000 tiles = 1000 × 2
Cost of 1000 tiles = Rs. 2000
Thus, the cost of 1000 tiles is Rs. 2000.
Question 13: One side of a square plot is 250m, find the cost of levelling it at the rate of Rs. 2 per square metre.
Solution 13:
Side of square plot = 250m
Area of Square = (side)2
Area of Square = (250)2
Area of Square = 250 × 250
Area of Square = 62500 m2
Cost of levelling = Rs. 2 per square meter
Cost of levelling 62500 m2 = 62500 × 2
Cost of levelling 62500 m2 = Rs. 1,25,000
Thus, the cost of levelling is Rs 1,25,000.
Question 14: The following figures have been split into rectangles. Find their areas. (The measures are given in centimetres)
Solution 14:
(i) Total Areas of the given figure,
Area of rectangle = L × B
Area of rectangle I = (2 × 4)
Area of rectangle I = 8cm2
Area of square = (Side)2
Area of square II = (3)2
Area of square II = (3 × 3)
Area of square II = 9 cm2
Area of rectangle = L × B
Area of rectangle III = (2 × 1)
Area of rectangle III = 2 cm2
Area of square = (Side)2
Area of square IV = (3)2
Area of square IV = (3 × 3)
Area of square IV = 9 cm2
Total area of the figure = Area of rectangle I + Area of Square II + Area of rectangle III + Area of square IV
Total area of the figure = 8 + 9 + 2 + 9
Total area of the figure = 28 cm2
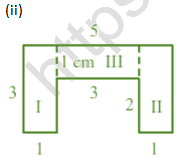
The given figure has three rectangles I, II and III.
Area of rectangle = L × B
Area of rectangle I = 3 × 1
Area of rectangle I = 3 cm2
Area of rectangle = L × B
Area of rectangle II = 3 × 1
Area of rectangle II = 3 cm2
Area of rectangle = L × B
Area of rectangle III = 3 × 1
Area of rectangle III = 3 cm2
Total area of the figure = Area of rectangle I + Area of rectangle II + Area of rectangle III
Total area of the figure = 3 + 3 + 3
Total area of the figure = 9 cm2
Question 15: Split the following shapes into rectangles and find the area of each. (The measures are given in centimetres)
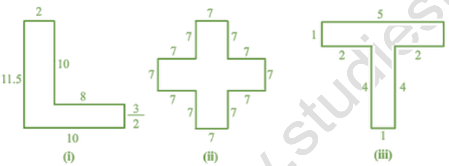
Solution 15:
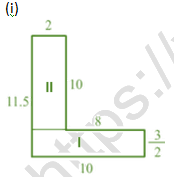
Area of rectangle = L × B
Area of rectangle I = 10 × 3/2
Area of rectangle I = 15 cm2
Area of rectangle = L × B
Area of rectangle II = 10 × 2
Area of rectangle II = 20 cm2
Total Area of the figure = Area of rectangle I + Area of rectangle II
Total area of the figure = 20 + 15 = 35 cm2
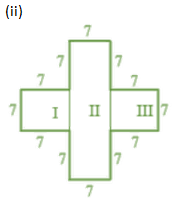
Area of square = (side)2
Area of square I = (7)2
Area of square I = 7 × 7
Area of square I = 49 cm2
Area of rectangle = L × B
Area of rectangle II = 21 × 7
Area of rectangle II = 147 cm2
Area of square = (side)2
Area of square III = (7)2
Area of square III = 7 × 7
Area of square III = 49 cm2
Area of the figure = Area of square I + Area of rectangle II + Area of square III
Area of the figure = 49 + 49 + 147
Area of the figure = 245 cm2

Area of rectangle = L × B
Area of rectangle I = 5 × 1
Area of rectangle I = 5 cm2
Area of rectangle = L × B
Area of rectangle II = 4 × 1
Area of rectangle II = 4 cm2
Total area of the figure = Area of rectangle I + Area of rectangle II
Total area of the figure = 5 + 4
Total area of the figure = 9 cm2
Question 16: How many tiles with dimensions 5 cm and 12 cm will be needed to fit a region whose length and breadth are respectively:
(i) 100 cm and 144 cm
(ii) 70 cm and 36 cm
Solution 16:
(i) Dimension of tiles = 5cm × 12cm
Dimensions of Region = 100cm × 144cm
Area of tile = 5 cm × 12 cm
Area of tile = 60 cm2
Area of region = 100 cm × 144 cm
Area of region = 14400 cm2
Number of tiles required = (Area of region)/(Area of one tile)
Number of tiles required = 14400/60
Number of tiles required = 240 tiles
(ii) Dimension of tiles = 5 cm × 12 cm
Dimensions of Region = 70 cm × 36 cm
Area of tile = 5 cm × 12 cm
Area of tile = 60 cm2
Area of region = 70 cm × 36 cm
Area of region = 2520 cm2
Number of tiles required = (Area of region)/(Area of one tile)
Number of tiles required = 2520/60
Number of tiles required = 42 tiles
Objective Type Questions ::->
Mark the correct alternative in each of the following:
Question 1: The sides of a rectangle are in the ratio 5 : 4. If its perimeter is 72 cm, then its length is
(a) 40 cm
(b) 20 cm
(c) 30 cm
(d) 60 cm
Solution 1: (b)
Let the Length and breadth of rectangle will be 5x and 4x respectively.
Perimeter of rectangle = 2 (Length + Breadth)
72 = 2 (5x+4x)
72 = 2 × 9x
72 = 18x
x = 72/18
x = 4
Thus, the length of the rectangle = 5x = 5 × 4 = 20 cm
Question 2: The cost of fencing a rectangular field 34 m long and 18 m wide at Rs 2.25 per meter is
(a) Rs. 243
(b) Rs. 234
(c) Rs. 240
(d) Rs. 334
Solution 2: (b)
Length = 34m
Breadth = 18m
Perimeter = 2 (Length + Breadth)
Perimeter of rectangle = 2 (34 + 18)
Perimeter of rectangle = 2 × 52
Perimeter of rectangle = 104m
Thus, the cost of fencing the field at the rate of Rs. 2.25 per meter = 104 × 2.25 = Rs. 234
Question 3: If the cost of fencing a rectangular field at Rs. 7.50 per meter is Rs. 600, and the length of the field is 24 m, then the breadth of the field is
(a) 8 m
(b) 18 m
(c) 24 m
(d) 16 m
Solution 3: (d)
Cost of fencing the rectangular field = Rs. 600
Rate of fencing the field = Rs. 7.50 per m
Perimeter of the field = (Cost of fencing)/(Rate of fencing)
Perimeter of the field = 600/7.50
Perimeter of the field = 80 m
Length of the field = 24 m
Perimeter of the field = L × B
80 = 2(24 + B)
80/2 = (24 + B)
40 = 24 + L
B = 40 - 24
Thus, breadth of the field is 16 m.
Question 4: The cost of putting a fence around a square field at Rs. 2.50 per meter is Rs. 200. The length of each side of the field is
(a) 80 m
(b) 40 m
(c) 20 m
(d) None of these
Solution 4: (c)
Cost of fencing the square field = Rs. 200
Rate of fencing = Rs. 2.50
Perimeter of the square field = (Cost of fencing)/(Rate of fencing)
Perimeter of the square field = 200/2.50
Perimeter of the square field = 80 m
Perimeter of square = 4(Side)
80 = 4(Side)
Side = 80/4
Side = 20m
Question 5: The length of a rectangle is three times of its width. If the length of the diagonal is 8√10m, then the perimeter of the rectangle is
(a) 15√10m
(b) 16√10m
(c) 24√10m
(d) 64m
Solution 5: (d)
ABCD is a rectangle.
Width of the rectangle BC = xm
Length of the rectangle AB = 3xm
ABC is a right angled triangle.
AC2 = AB2 + BC2
640 = (3x)2 + x2
640 = 9x2 + x2
640 = 10x2
x2 = 640/10
x = 64
x = √64
x = 8 m
Breadth of the rectangle x = 8 m
Length of the rectangle 3x=3×8
Length of the rectangle=24 m
Perimeter of rectangle = 2 (L + B)
Perimeter of rectangle = 2 (24 + 8)
Perimeter of rectangle = 2 × 32
Perimeter of rectangle = 64 m
Question 6: If a diagonal of a rectangle is thrice its smaller side, then its length and breadth are in the ratio
(a) 3: 1
(b) √3: 1
(c) √2 : 1
(d) 2√2: 1
Solution 6: (d)
Smaller side of the rectangle BC = x
Length of the larger side AB = y
It is given that the length of the diagonal is three times that of the smaller side.
Diagonal of the rectangle 3x = AC
By using Pythagoras theorem
Question 7: The ratio of the areas of two squares, one having its diagonal double than the other, is
(a) 1: 2
(b) 2: 3
(c) 3: 1
(d) 4: 1
Solution 7: (d)
Let ABCD and PQRS as the two squares.
The diagonal of square PQRS is twice the diagonal of square ABCD.
PR = 2AC
Question 8: If the ratio of areas of two squares is 225 : 256, then the ratio of their perimeters is
(a) 225 : 256
(b) 256 : 225
(c) 15 : 16
(d) 16 : 15
Solution 8: (c)
Let ABCD and PQRS as the two squares.
Let the lengths of each side of ABCD and PQRS be x and y.
Question 9: If the sides of a square are halved, then its area
(a) remains same
(b) becomes half
(c) becomes one fourth
(d) becomes double
Solution 9: (c)
Question 10: A rectangular carpet has area 120 m2 and perimeter 46 meters. The length of its diagonal is
(a) 15 m
(b) 16 m
(c) 17 m
(d) 20 m
Solution 10: (c)
Question 11: If the ratio between the length and the perimeter of a rectangular plot is 1: 3, then the ratio between the length and breadth of the plot is
(a) 1: 2
(b) 2: 1
(c) 3: 2
(d) 2: 3
Solution 11: (b)
Question 12: If the length of the diagonal of a square is 20 cm, then its perimeter is
(a) 10√2cm
(b) 40 cm
(c) 40√2cm
(d) 200 cm
Solution 12: (c)

