


Please refer to link below to download pdf file ofCBSE Class 10 Arithmetic Progressions HOTs



ADDITIONAL QUESTION
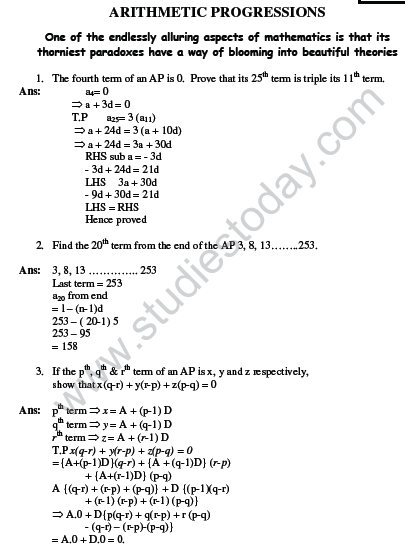
1. The angles of a triangle are in A.P. If the greatest angle equals to the sum of the other two, find the angles.
2. If first, second and the last terms of an A.P. are a, b, 2a respectively then, find sum of all the terms.
3. Find the sum of n terms of a series √2 + √8 + √18 + . . .
4. Which term of the A.P. 7, 12, 17, 22,…..is 30 more than 20th term?
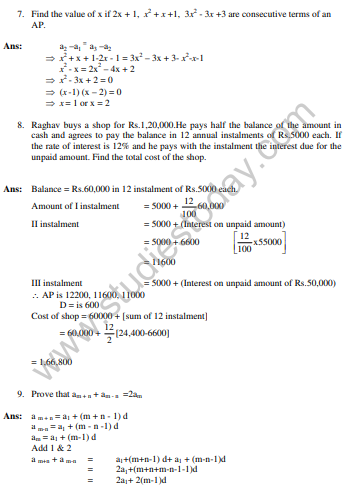
5. 5 times of the 5th term of an AP is equal to 12 times of its 12th term. Find the 17th term.
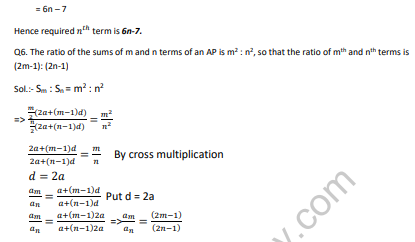
6. Find three terms in A.P. whose sum is 15 and whose product is 105
7. Find the sum of all the numbers between 200 and 500 which are divisible by 7.
8. If the sum of n terms of an AP is 3n2+5n, then which of its term is 164?
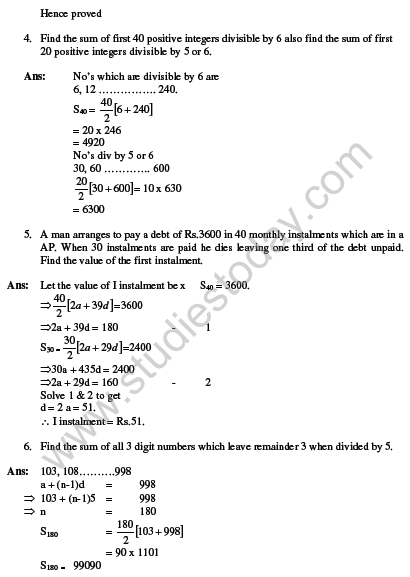
Please refer to link below for CBSE Class 10 Mathematics HOTs Arithmetical Progression Set A






Please refer to attached file for CBSE Class 10 Mathematics HOTs Arithmetic Progressions.
ARITHMETIC PROGRESSION
1 MARK
1) Which term of the sequence 4,9,14...is 124?
2) Find the 10th term from the end of A.P 3, 8, 13, 18...253
3) For what value of K, the number x,2x+k,3x+6,are three consecutive terms of A.P
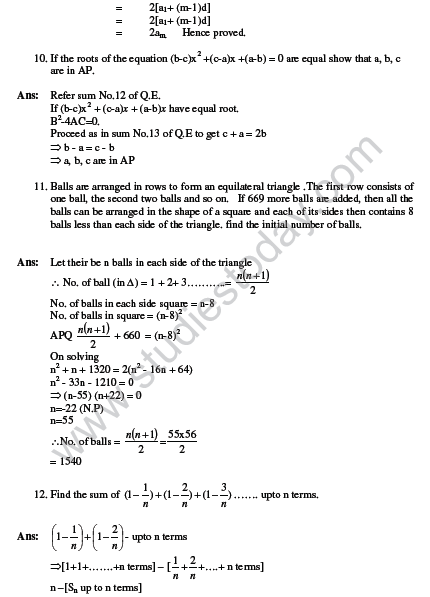
4) How many numbers of two digits are divisible by 8?
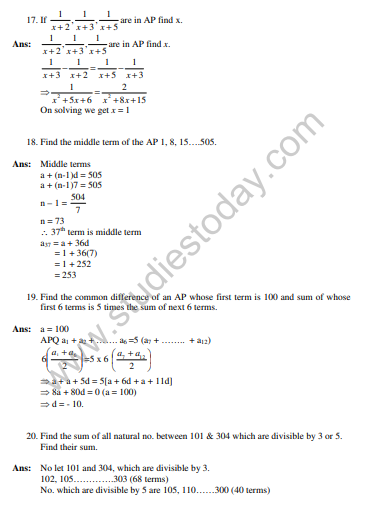
5) Find the middle term of A.P 1,8,15,...,505
6) Write next term of A.P ∫8, ∫18, ∫32....
7) What is the common difference of an A.P in which a23 -a18 =45
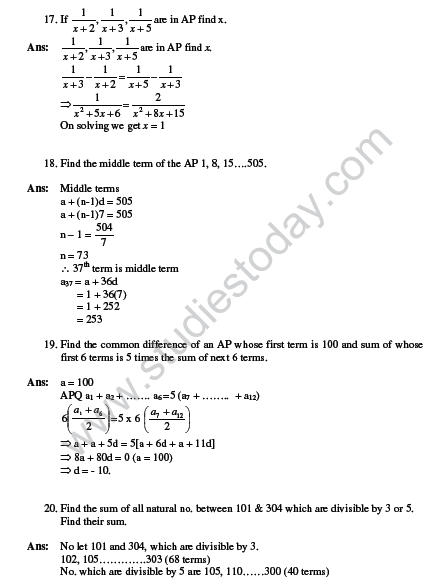
8) Find first three terms of an A.P whose nth term is -5 + 2x
9) In the given A.P, find the missing terms 0,_,-8,-12,_.
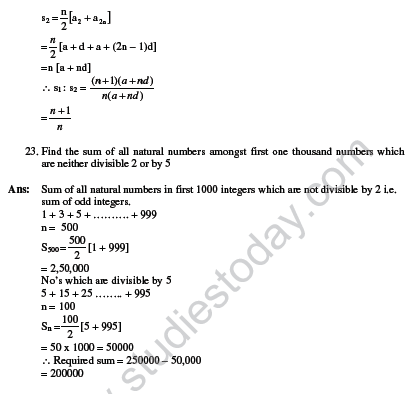
10) Find the sum of all odd integers between 1 and 100 which are not multiples of 4
2/3 MARKS
Q.1 How many terms of A.P 18, 16, 14...Should be taken so that their sum is zero?
Q.2 If the 10th term of an A.P is 47 and first term is 2, find the sum of the first 15 terms
Q.3 Solve the equation-2+5+8...+x=155
Q-4 Which term of A.P 121,117,113....is the first negative term?
Q-5 How many multiples of 4 lie between 10 and 250?
Q-6 If the sum of n terms of an A.P is n2 +2x, find the A.P and the 20th term.
Q-7 For what values of n, nth term of the series 3, 10, 17...and 63,65,67...are equal?
Q-8 If seven times the seventh term of an A.P is equal to 11 times the eleventh term, show that 18th term of an A.P is zero.
Q-9 If 9th term of an A.P is 0, prove that 29th term is double of the 19th term
Q-10 Determine the A.P whose zero term is 16 and when 5th term is subtracted from 7th ,we get 12
Answers
1 mark
a. n=25
b. 209
c. k=3
d. n=11
e. middle term=73
f. 5√2,6√2,7√2
g. d=9
h. –3,-1,1
i. 0,-4,-8,-12,-16
j. 2500
2/3 marks
1. 19
2. 255
3. 29
4. 32nd term
5. 60
6. 3,5,7,9
T20 =41
7. n=13
8. a+17d=0
T18 =0
10. 4,10,16,22
MORE QUESTIONS
Arithmetic Progressions
Key points
1. SEQUENCE : - A set of numbers arranged in some definite order and formed according to some rules is called a sequence.
2. PROGRESSION :- The sequence that follows a certain pattern is called a progression.
3. ARITHMETIC PROGRESSION :- A sequence, in which the difference obtained by subtracting from any term its preceding term is consistent throughout, is called an arithmetic sequence or arithmetic progression (A. P.). The general form of an A.P. is a, a+d, a+2d, . . . .
4. GENERAL TERM :- If 'a' is first term and 'd' is common difference in an A.P. then nth term (general term) is given by an = a + (n –1)d .
5. SUM OF n TERMS OF AN A.P. :- Sum of first n terms of an A.P. is given by :-
Sn = n/2 {2a+ ( n–1) d}
where, a : first term, d: common difference.
If l is the last term of a finite A.P., then the sum is given by Sn =n/2 {a+l }
6. (i) If an is given, then common deference d = an– an-1
(ii) If Sn is the sum of n terms of an A.P., then nth term is given by an = Sn - Sn-1
(iii) If a sequence has n terms, its rth term from the end = its ( n-r +1)th term from the beginning.
(iv) If a, b, c are in A.P., then b= a+c/2
Questions
1 mark question (Question No. 16 to 20 are under HOTS) :-
Q. 1 Write 17th term of the A.P. 3, 7, 11, . . . . . . . .
Ans.1. 67
Q. 2 nth term of a sequence is 3 – 5n. Is it A.P.?
Ans.2. Yes
Q. 3 If nth term of an A.P. is 2n+5, write the common difference of this A.P.
Ans.3. 2
Q. 4 Which term of –3, 1. 5, . . . . . . . is 69?
Ans.4. 19th term
Q. 5 If 3, 2k+3, 9 are in A.P., then write the value of 'k'.
Q. 6 Is –10, a term of 28, 25, 22, . . . . . . .?
Ans.6. No
Q. 7 Write first term and common difference of the A.P. 3.3, 3.1, 2.9, . . . . . . . .
Ans.7. first term = 3.3, common difference = –0.2
Q. 8 Write nth term of –5, 1, 7, . . . . . . . .
Ans.8. 6n –11
Q. 9 The first term of an A.P. is 9 and fifth term is 14. Write common difference of the A.P.
Q. 10 Write first four terms of an A.P. Whose second term is –4 and common difference is –1.
Ans.10. –3, –4, –5, –6.
Q. 11 Which term of 11, 8, 5, . . . . . . is –16?
Ans.11. 10th term
Q. 13 Write an A.P., whose nth term is 5n – 7.
Ans.13. –2, 3, 8, . . . . . .
Q. 14 Write the sum of three terms of the A.P. 3, 7, 11, . . . . . .
Ans.14. 15, 19, 23
Q. 15 Write the sum of first 10 natural numbers.
Ans.15. 55
Q. 16 The sum of 6th and 7th term of an A.P. is 39 and common difference is 3. Write its 6th term.
Ans.16. 18
Q. 17 The sum of 3 numbers in A.P. is 30. If the greatest number is 13, write common difference.
Ans.17. 3
Q. 18 Write 11th term from the end of the A.P. 3, 7, 11, 15, . . . . . . . . ., 143.
Ans.18. 103
Q. 19 For what value of 'x' the numbers 1/2x , 1/x , x+1/2x are in A.P.? (x ≠ 0)
Ans.19. x = 2
Q. 20 Write the sum of first 10 odd natural numbers.
Ans.20-. 100
2 marks questions (Question no. 36 to 40 under HOTS)
Q. 21 How many terms of A.P. 22, 20, 18, . . . . . . . . should be taken so that their sum is zero?
Ans.21. 23
Q. 22 Find the sum of odd positive integers less than 199.
Ans.22. 9801
Q. 23 How many two digits numbers between 3 and 102 are divisible by 6?
Ans.23. 15
Q. 24 If 7 times the 7th term is equal to 11 times the 11th term of an A.P. Find its 18th term.
Ans.24. 0
Q. 25 Which term of A.P. 13, 21, 29, . . . . . . will be 48 less than its 19th term?
Ans.25. 13th term
Q. 26 Find the A.P. whose 3rd term is –13 and 6th term is +2.
Ans.26. –23, –18, –13, - - -
Q. 27 Find the A.P., whose 5th term is 23 and 9th term is 43.
Ans.27. 3, 8, 13 - - -
Q. 28 The angles of a triangle are in A.P. If the smallest angle is one fifth the sum of other two angles. Find the angles.
Ans.28. 300, 600 and 900
Q. 29 Aditi saved Rs. 500 in the first month of a year and then increased her monthly savings by Rs. 50. If in the nth month, her monthly savings become Rs 1000. Find the value of 'n'.
Ans.29. n =11
Q. 30 The sum of first n terms of an A.P. is 2n2 + n . Find nth term and common deference of the A.P.
Ans.30. nth term = 4n–1
common differnece = 4
Q. 31 Which term of the A.P. 38, 35, 32, . . . . . . is the first negative term.
Ans.31. 14th term {Hint an <0 }
Q. 32 The sum of 3rd and 7th terms of an A.P. is 14 and the sum of 5th and 9th terms is 34. Find the first term and common difference of the A.P.
Ans.32. First term = –13
common difference = 5
Q. 33 Find the A.P. whose second term is 10 and the 6th term exceeds the 4th term by 12.
Ans.33. 4, 10, 16, 22, - - -
Q. 34 Sarthak starts a game and scores 100 points in the first attempt and he increases the points by 5 in each attempt. How many points will he score in the 30th attempt.
Ans.34. 245
Q. 35 If –9, –14, –19, – 24, − − − − − is an A.P. , then find a30 – a20 .
Ans.35. –50
Q. 36 Find the 19th and 20th terms of the sequence defined by n2 when n is even
n2+1 when n is odd
⎨
Ans.36. 363 and 400
.
Q. 37 The fourth term of an A.P. is equal to 3 times the first term and the seventh term exceeds twice the third term by 1. Find the first term and the common difference of the A.P.
Ans.37. first term = 3
common difference = 2
Q. 38 Find an A.P. of 8 terms whose first term is 1/2 and last term is 17/6 .
Ans.38. 1/2, 5/6, 7/6 ----
Q. 39 For an A.P. a1 , a2 , a3 ,......., if a4 / a1 = 2/3 , then find a6 / a8
Ans.39. 4/5 (Hint : a +3d / a +6d = 2/3)
Q. 40 2nd , 31st and last terms of an A.P. are 31/4, 1/2 and – 13/ 2 respectively. Find the number of terms in the A.P.
Ans.40. 59
3 marks questions (Question no. 56 to 60 under HOTS)
Q. 41 Find the sum of the first 30 terms of an A.P., whose nth term is 2–3n.
Ans.41. –1335
Q. 42 Find the sum of A.P. :- 6+10+14+.......+102?
Ans.42. 1350
Q. 43 If mth and nth terms of an A.P. are 1/n and 1/m respectively, then find the sum of mn terms.
Q. 44 Find the sum of all the three digits numbers each of which leaves the remainders 3 when divided by 5.
Ans.44. 990 90
Q. 45 A picnic group consists of students whose ages are in A.P., the common difference being 3 months. If the youngest student is just 7 years old and the sum of ages of all the students is 250 years. Find the number of students in the group.
Ans.45. 25 students
Q. 46 If nth term of an A.P. is 4, common deference is 2 and sum of n terms is –14, then find first term and numbers of terms.
Ans.46. a = –8, total term = 7
Q. 47 The sum of first six terms of an A.P. is 42. The ratio of the 10th term to the 30th term is 1:3. Find first term and 11th term of the A.P.
Ans.47. a = 2
11th term = 22
Q. 48 The sum of n terms of two A.P's. are in the ratio 3n +8: 7n +15 . Find the ratio of their 12th terms.
Ans.48. 7:16
Q. 49 The sum of three numbers in A.P. is 24 and their product is 440. Find the numbers.
Ans.49. 5, 8, 11
Q. 50 The sum of first 16 terms of an A.P. is 528 and sum of next 16 terms is 1552. Find the first term and common difference of the A.P.
Ans.50. first term = 3
common difference = 4
Q. 51 Find the number of terms of the A.P. 57, 54, 51, . . . . . so that their sum is 570. Explain the double answer.
Ans.51. 19 or 20 ( 20th term is zero)
Q. 52 If the sum of first 20 terms of an A.P. is one third of the sum of next 20 terms. If first term is 1, then find the sum of first 30 terms.
Ans.52. 450
Q. 53. The digits of a three digit positive number are in A.P. and the sum of digits is 15. On subtracting 594 from the number the digits are inter changed, find the numbers.
Ans.53. 852
Q. 54 The sum of first 8 terms of an A.P. is 140 and sum of first 24 terms is 996. Find the A.P.
Ans.54. 7, 10, 13, - - -
Q. 55 If mth , nth and rth terms of an A.P. are x, y and z respectively, then prove that :- m( y – z) + n(z – x) + r (x – y) = 0
Ans.55. (Hint : ( an = a+(n-1)d)
Q. 56 The sum of 5th and 9th terms of an A.P. is 8 and their product is 15. Find the sum of first 20 terms of the A.P.
Ans.56. 115, 45, { d = ±1/2}
Q. 58 Balls one arranged in rows to form an equilateral triangle. The first row consists of one ball, the second of two balls, and so on. If 669 more balls are added, then all the balls can be arranged in the shape of a square and each of its sides then contains 8 balls less than each side of the triangles. Determine the initial number of balls.
Ans.58. 1540 balls
Q. 59 If the roots of the equation a(b – c) x2 + b(c – a) x + c (a – b) = 0 are equal, then show that 1/a , 1/b , 1/c are in A.P.
Q. 60 If the sum of m terms of an A.P. is n and the sum of n terms is m, then show that sum of (m+n) terms is – (m+n).

